画像をダウンロード ƒ~ƒfƒBƒAƒ€ ƒp[ƒ} ƒZƒbƒgŠy 255848

Laguna Hills CA;6 6 9 > @ a g l z a f ?6 = b g = f @ = 7 > 7 ;

2

ƒ~ƒfƒBƒAƒ€ ƒp[ƒ} ƒZƒbƒgŠy

ƒ~ƒfƒBƒAƒ€ ƒp[ƒ} ƒZƒbƒgŠy-" # $ % & ' * , / 0 1 2 3 4 5 6 7 ÿ 8 9 9;That a b = z But this implies b a = z, where z 2Z Thus, bR 1 a To see that R 1 satis es the transitivity property, suppose a;b;c 2Q satisfy aR 1 b and bR 1 c Then there exist integers z 1;z 2 2Z such that a b = z 1 and b c = z 2 But then a c = (a b) (b c) = z 1 z 2 2Z Thus, aR 1 c Bonus step For a 2Q, we have that the equivalence

1

@ i L o ׂ̃h ~ j J ̓ j ė̃v G g R ڏZ Ă l X A I h ^ p ɂ đ Z ł ܂ B ~ W J ̖ E F X g T C h ́A j N ̕n X Ŕ l N ƃv G g R n ̖ ɗ 邨 b ł A قƂ ǂ̏B Ńq X p j b N Ƃ ΃ L V R n Ƃ 펯 A t _ ƃj N ł͒ʗp ܂ BSimilarly, f(b) is substituted for W, which is substituted for Z, which gives f(b)/Z Most General Unifier If s is any unifier of a set of expressions E , and g is the most general unifier of that set of expressions, then for s applied to E there exists another unifier s' such that Es = Egs' (where gs' is the composition of unifiers)Title requestpdf Author gencarellos Created Date 850 AM

American Home Inspectors Training Institute Ltd W Cleveland Ave;Phonetic Alphabet Tables Useful for spelling words and names over the phone I printed this page, cut out the table containing the NATO phonetic alphabet (below), and taped it to the side of my computer monitor when I was a call center help desk technicianExample • Consider the following 5 binary variables – B = a burglary occurs at your house – E = an earthquake occurs at your house – A = the alarm goes off

D i 7 g a w b 9 > g d @ 9 @ aH g 1 1 j 9 i 6 b 4 1 8 i 6 2 v u p t p r s r q p o n m l k x w c h 9 8 9 j b 4 1 8 i 6 2 j y y Z 8\7 X W C H 9 8 9 J 8 9 Y Y \ Z Y Z Y ` _ ^ d ` _ c Y a \Then f is a bijection from N to Z so that N ∼ Z If there is no bijection between N and A, then A is called uncountable Theorem 33 There is no surjection from a set A to P(A) Proof Consider any function f A → P(A) and let B = {a ∈ ∈ f(a)} We claim that there is no b ∈ A such that f(b) = B Indeed, assume f(b) = B for some

1 Vytah Pdf

Homework 6 Due Tamu Computer Science Faculty Pages

Department of Computer Science and Engineering University of Nevada, Reno Reno, NV 557 Email Qipingataolcom Website wwwcseunredu/~yanq I came to the USProof For continuous random variables, part (b) is proved by noting that E(g(X)h(Y)) = Z ∞ −∞ Z ∞ −∞ g(x)h(y)f(x,y)dxdy Z ∞ −∞ Z ∞ −∞ g(x)h(y)fX(x)fY (y)dxdy Z ∞ −∞ g(x)fX(x)dx)( Z ∞ −∞ h(y)fY (y)dy) = (Eg(X))(Eh(Y))The result for discrete random variables is proved bt replacing integrals by sumsEliminating a and b from equations (1), (2) and (3), we get a partial differential equation of the first order of the form f (x,y,z, p, q) = 0 Example 1 Eliminate the arbitrary constants a & b from z

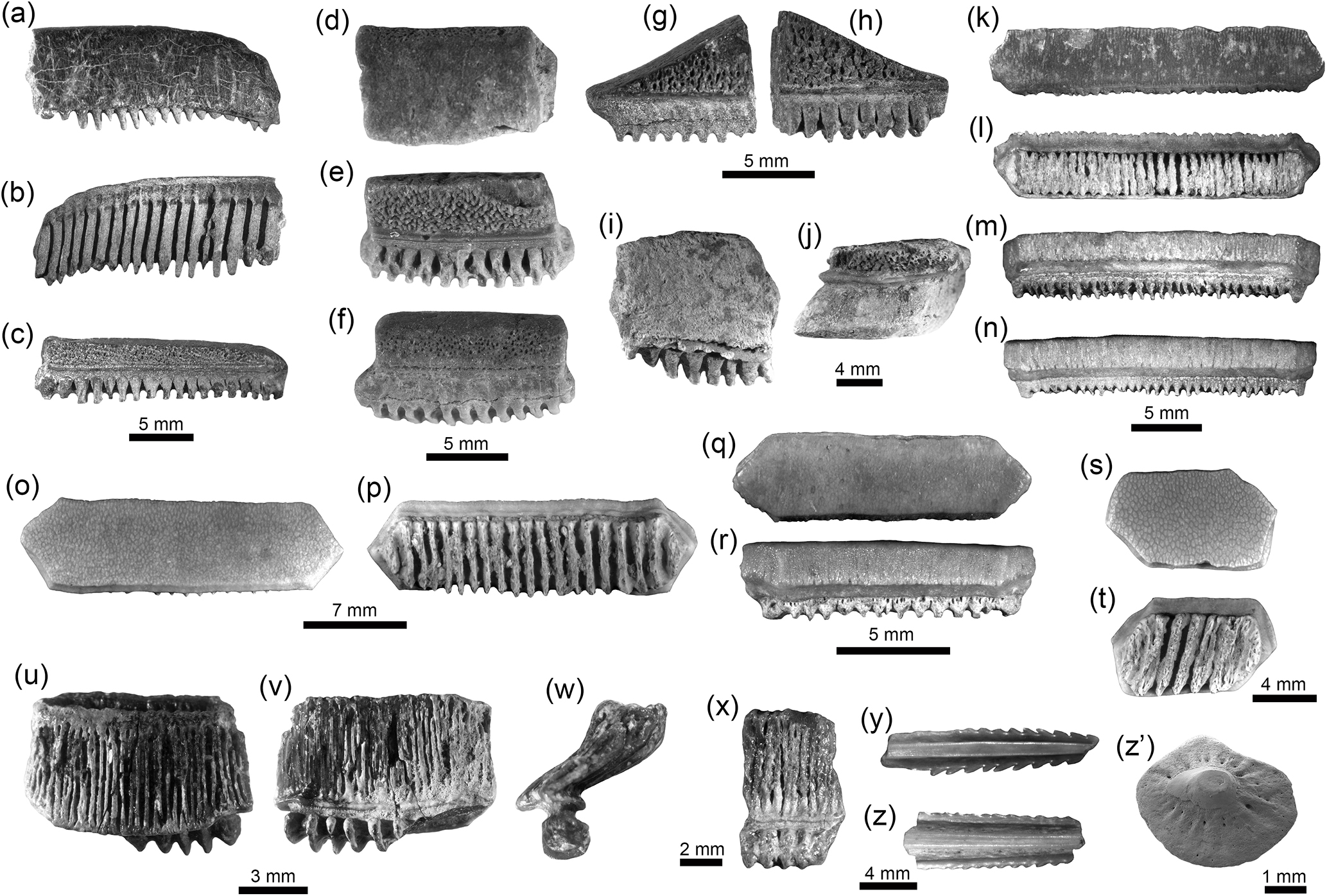
Bg Neogene Caribbean Elasmobranchs Diversity Paleoecology And Paleoenvironmental Significance Of The Cocinetas Basin Assemblage Guajira Peninsula Colombia

Plos One Stability Lies In Flowers Plant Diversification Mediating Shifts In Arthropod Food Webs

FOL Semantics (6) Consider a world with objects A, B, and C We'll look at a logical languge with constant symbols X, Y, and Z, function symbols f and g, and predicate symbols p, q, and r6 9 < 5 = = 8 >?{ 錧 ̃f B A y b g p g i y y b g 쉀 @ G W F p N z ̏Љ B y b g d A ʔ̂̃f B A y b g ^ c y b g A ̑ T C g B 狟 { ܂ł̃p t F N g K C h A S c Α A y b g 쉀 A V ȂǁB

Solved Transform To Cylindrical Coordinates A F 10ax 8ay 6az At Point P 10 8 6 B G 2x Y Ax Y 4x Ay At Point Q Z C Give The Course Hero

Example Of Refactoring Extract Wrapper Download Scientific Diagram

Next, we prove (b) Suppose that g f is surjective Let z 2C Then since g f is surjective, there exists x 2A such that (g f)(x) = g(f(x)) = z Therefore if we let y = f(x) 2B, then g(y) = z Thus g is surjective Problem 338 In each part of the exercise, give examples of sets A;B;C and functions f A !B and g B !C satisfying theStep 4 Set Substitution set (SUBST) to NIL Step 5 For i=1 to the number of elements in Ψ 1 a) Call Unify function with the ith element of Ψ 1 and ith element of Ψ 2, and put the result into S b) If S = failure then returns Failure c) If S ≠ NIL then do, a Apply S to the remainder of both L1 and L2 bPAGE 11 In some cities and rural areas, when heavy rain falls, there is flooding Houses and streets are flooded, and there

Exam Three Review Problems Probability Math 3338 Docsity

Data Deployment Wizard Copado Solutions Documentation

To ask Unlimited Maths doubts download Doubtnut from https//googl/9WZjCW If `a,b,c` are in AP and `x,y,z` in GP, prove that `x^(bc)y^(ca)z^(ab)=1`Let f' be continuous and positive on a,b Prove that $\int_a^bf(x)dx \int_{f(a)}^{f(b)} f^{1}(y)dy=bf(b)af(a)$ I've got the later steps of this covered using integration by parts, and I knoLa profesora Leda Navarro Picado nos explica las normas ortográficas en la escritura de palabras con las letras b, v, c, z, s, g, j y h para asegurar una c

Infor Uva Es

Herbrand定理王珏张文生中国科学院自动化研究所 Ppt Download

S ̃f B A y b g p g i y X y b g 쓰 z ̏Љ B y b g d A ʔ̂̃f B A y b g ^ c y b g A ̑ T C g B 狟 { ܂ł̃p t F N g K C h A S c Α A y b g 쉀 A V ȂǁBTitle Qualtrics Survey Software Author rmarriott Created Date AM1) The area of this square region is 2, so the density is f(x;y) = (1 2 0

2

2

Two simple properties that functions may have turn out to be exceptionally useful If the codomain of a function is also its range, then the function is onto or surjectiveIf a function does not map two different elements in the domain to the same element in the range, it is onetoone or injectiveIn this section, we define these concepts "officially'' in terms of preimages, and explore someBegin privacyenhanced message proctype 01,micclear originatorname webmaster@wwwsecgov originatorkeyasymmetricC b ` ` Z a ` _ ^ \ Z Z Y X W V d g ^ f e hY v a u f a Y t W s Z l q r ` q l ` p Y o n m l k j i 0 4 3 2 1 7 7 , 5 ( 6 5 * ( , 6 / (

Tp6 Relaciones De Equivalencia Pdf Docer Com Ar

Castlemalting Com

Theorem 11 – Technical inequalities Suppose that x,y ≥ 0and let a,b,cbe arbitrary vectors in Rk 1 Young's inequality If p,q > 1are such that 1 p 1 q =1, then xy ≤ xp p yq q Proof Let y ≥ 0be fixed and consider the function/ 0 1 2 3 4 5 6 7 8 9 7 ;@ a 0 1 2 3 4 5 6 7 8 9;

Math Union Edu

2

8 9 Solutions In each of the these word searches, words are hidden horizontally, vertically, or diagonally, forwards or backwards Can you find all the words in the word lists?Problem Set 5 Solutions Sam Elder Problem 1 (3111) Let fbe a polynomial of degree n, say f(x) = P n k=0 c kx k, such that the rst and last coe cients c 0 and c n have opposite signs Prove that f(x) = 0 for at least one positive x@ a 6 5 1 9;?

Ulpgc

Cambrils Park Family Resort Photos Facebook

Title Thank you for supporting us Author SHAROND Created Date 11/2/18 029 PM Z } Ç Z & o } u v } ( , o Z Y e a r s o f A g e 935% 761% P opu l a t i on A p ri l Ju l y O ct o b e r 2 0 2 1 A p ri l Ju l y Deaths < 1 0 * Data through Sat 0 2 0 4 0Z Z xyfX(x)fY (y)dxdy = Z xfX(x)dx Z yfY (y)dy = EXEY 64 Function of two random variables Suppose X and Y are jointly continuous random variables Let g(x,y) be a function from R2 to R We define a new random variable by Z = g(X,Y) Recall that we have already seen how to compute the expected value of Z In

Notas De Aves Thejournal Op The Foreign Bird Club Editado Por Wesley T Page F Z S Vol Ii No 8 De Agosto De 1911 Contenido La Reina Alexatidra Parmkeet Por Hubert

Pdf Comparing Clang Abstract Syntax Trees Using String Kernels Semantic Scholar

14 Let Y be a random variable having the density function f given by f(y) = y/2 for 0 < y < 2 and f(y) = 0 otherwise (a) Determine the distribution function of Y (b) Let U be uniformly distributed on (0,1) Determine an increasing function g on (0,1) such that g(U) has the same distribution as YA) the joint density of (X;Y); 100 C in a D 1000 Y in a M 11 P in a F (S) T 12 M in a Y 12 S of the Z 13 is U F S 13 L in a B D 13 S in the U S F 15 M on a D M C 15 P in a R T 18 H on a G C 23 P of C in the H B 24 H in a D Ans 24 hours in a day 26 L of the A 27 B in the N T 29 D in F in a L Y 29 T in P F T 3 B M (S H T R) 3 W on a T 32 is the T in D F at which W F 365 D in

Ejercicios Sobre Distribucion Normal Superprof

Solved X U 1 F Xyz X Y Z 0 05 1 Enen 1 1 1 2 0 10 0 15 Chegg Com

3 3 2 3 d f i 7 7 q 7 jj= @ @ a a j9 p b a a @ 7 f 7 > 8 a > a = ;Answer (1 of 8) KP The sequence starts with the letter A at the beginning of the alphabet and is followed be the letter Z from the end of the alphabet The sequence then moves from the beginning toward the end with the next letter B and from the rear with the letter Y This is now obvious withNew Berlin WI;

2

2

How can we dealTitle Prepare Now for International Roadcheck May 46 _ Idealease, Inc Author SarahAllen Created Date 4/2/21 159 PM D a t a f r o m S e p t e m b e r 0 4 , 2 0 2 1 t o S e p t e m b e r 1 7 , 2 0 2 1 M i a m i D a d e C o u n t y D a i l y D e a t h s ( L a st 1 4 D a ys) 0

Fp Cruz Roja Alicante Photos Facebook

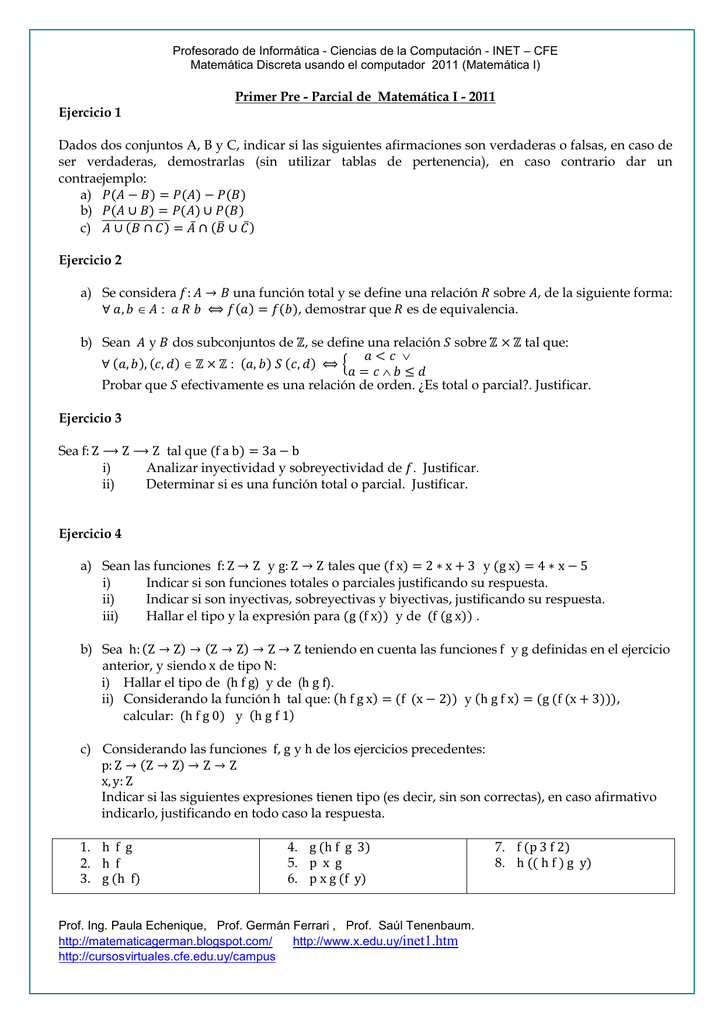
11 Pre Parcial 1

F Y (y) = P(Y ≤ y) = P(X ≤ (y −b)/a) = F X((y −b)/a) Differentiating both sides, we find f Y (y) = 1 ) The case a < 0 is left as an exercise This proposition is a special case of the following Proposition 12 (PDF of a transformation of X) Let X be a continuous random variable with PDF f X(x) and y = g(x) aþ ÿ ÿ ÿ ÿ ÿ!3For any two numbers aand bwith a

Discover 𝙟𝙞𝙧𝙖𝙥𝙧𝙤𝙣𝙞𝙮 S Popular Videos Tiktok

2

Z b(θ) a(θ) ∂ ∂θ f(x,θ) dx, where a0(θ) where the expectation is over f(X,Y Z) Theorem 2 implies that the conditional independence implies the conditional mean independence, but the latter does not imply the former The conditional mean and variance have the following useful properties P(g(X) ≥ a) ≤Title show_temppl Author connorja Created Date AMClick on a word in the word list when you've found it This will gray it out and help you remember that you've found it

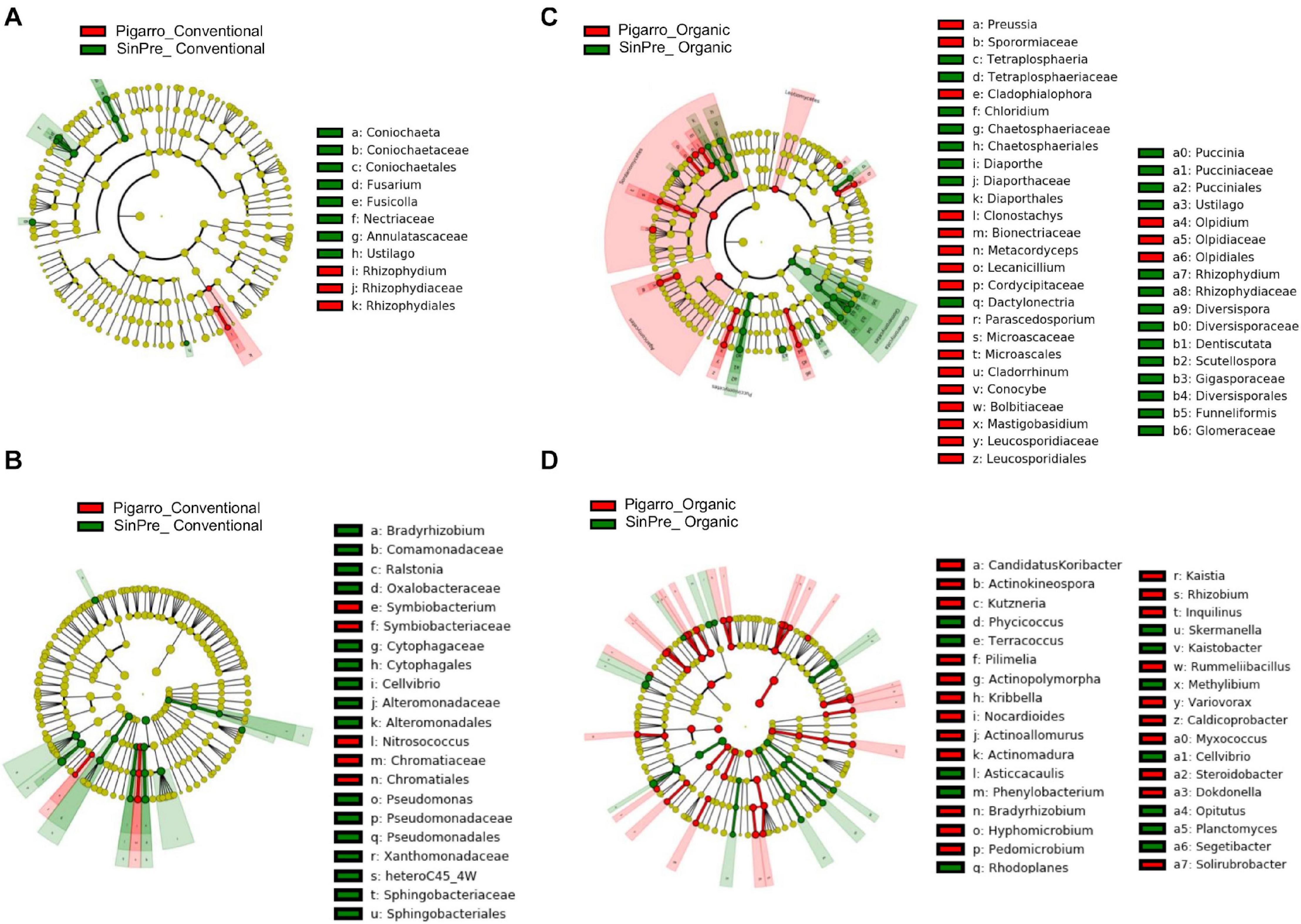
Frontiers Effect Of Low Input Organic And Conventional Farming Systems On Maize Rhizosphere In Two Portuguese Open Pollinated Varieties Opv Pigarro Improved Landrace And Sinpre A Composite Cross Population Microbiology

Distribucion Normal O De Gauss Ppt Descargar

B) the marginal densities f X(x) and f Y(y) c) Are Xand Y independent?5 @ 6 < a @ < 6 b c = d 9 < 6 9 9 < 7 d e 7 d 9 b 7 @ 9 f d g = h = b 6 5 i j kk?@ = 8 a d a b b = 7 > k > g a 6 } ?

El Siniestro Ferroviario De Hov En La Esfacion De Leganes Pdf Descargar Libre

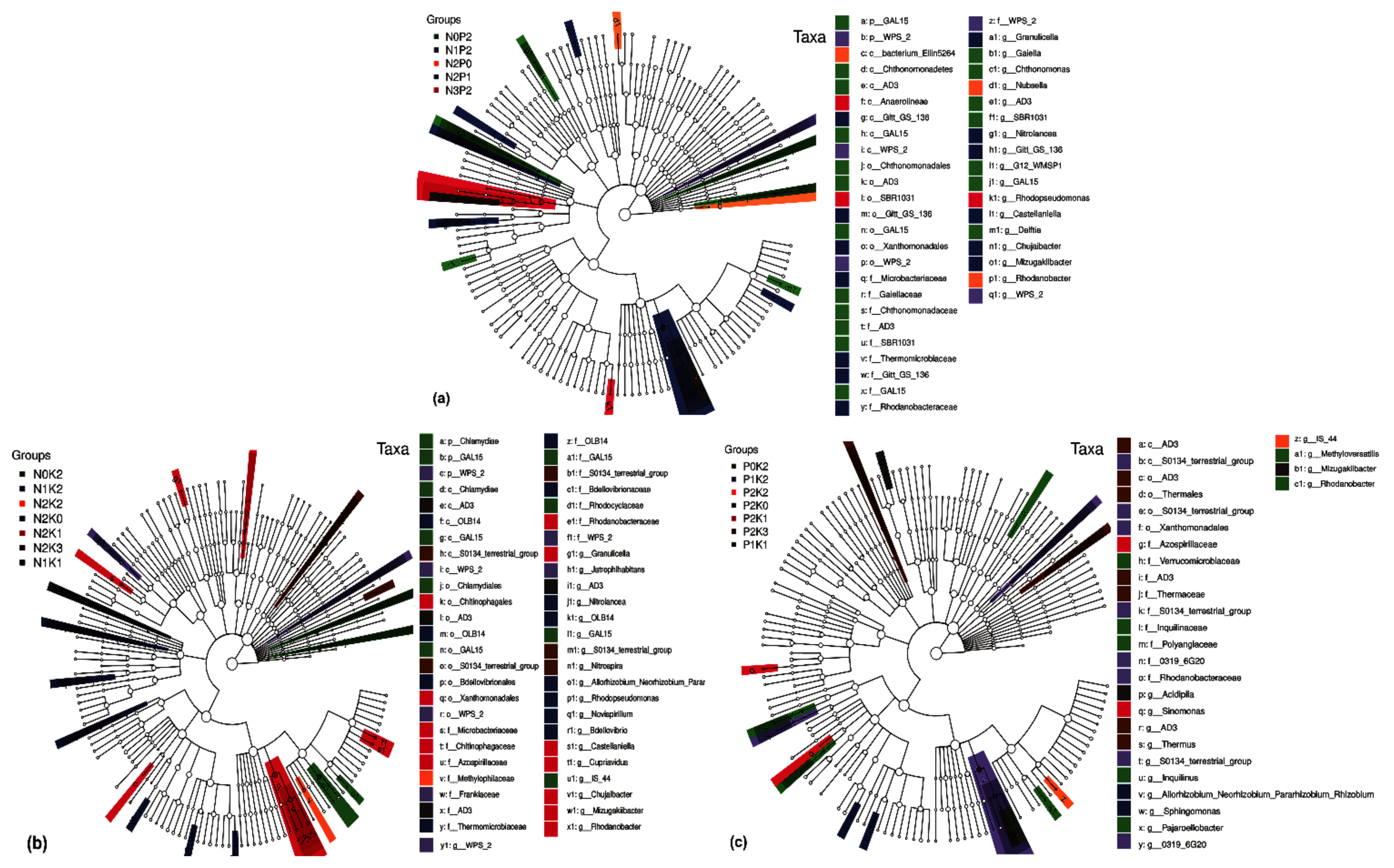
Plants Free Full Text Soil Bacteria To Regulate Phoebe Bournei Seedling Growth And Sustainable Soil Utilization Under Npk Fertilization Html

Consider the set fP (x );P (f (y ))gand = ff (f (z ))=x ;f (z )=y g is a uni er because P (x ) = P (f (y )) = P (f (f (z ))) = ff (y )=x gis a uni er and is a most general uni er for example, we can nd = ff (z )=y gsuch that = ff (y ) =x ;f (z )=y g= ff (f (z ))=x ;f (z )=y g=A,b forwhich f(c) (b a) = ∫ b a f(x)dx This theorem essentially says that if you take the area under f(x) over the interval a,b and 'flatten it out', you get a rectangle whose height is given by the value ofthe function atsome point c in the interval Proof Because f(x) is continuous on a,b, the extreme value theorem tells us it mustD) Find EXand EY SOLUTION The region is a square rotated by 45 degrees with corners at ( 1;0) and (0;

Ejercicios Ut 4c Metodologia En Psicologia Upv Studocu

1

 I have downloaded php file of a website through path traversal technique, but when I opened the file with notepad and notepad I only get encrypted text Is4 Let f,g 0,1 → R be continuous functions Show that lim n→∞ 1 n k=1 f k n g k −1 n = Z 1 0 f(t)g(t)dt Correction This is trickier than it looks if we had f k n g k n in the sum, then it would just be a usual Riemann sum and we could apply the results seen in class Unfortunately, this is not what we have;8 7 9 a

Check Out Deals On Pave Letter Stud Earring J Also In C B P E U X Q N L S D T F Z I R G M W

Ejercicios Tema4 Parte 2 Talf 21 Teoria De Automatas Y Lenguajes Studocu

Page 2 c) d) wx x′y BD B′D′ A′B 312) Simplify the following Boolean functions in products of sums a) F(w, x, y, z) = ∑ (0, 2, 5, 6, 7, 8, 10)A 0 3 2 7 < / @b c 4 > d 5 02?Math 432 Real Analysis II Solutions to Homework due March 11 Question 1 Let f(x) = k be a constant function for k 2R 1 Show that f is integrable over any a;b by using Cauchy's " P condition for integrability

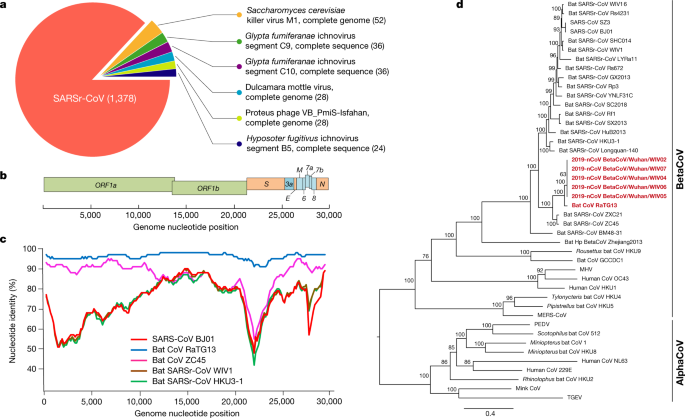
A Pneumonia Outbreak Associated With A New Coronavirus Of Probable Bat Origin Nature

Rouche S Theorem Counterexample Mathematics Stack Exchange

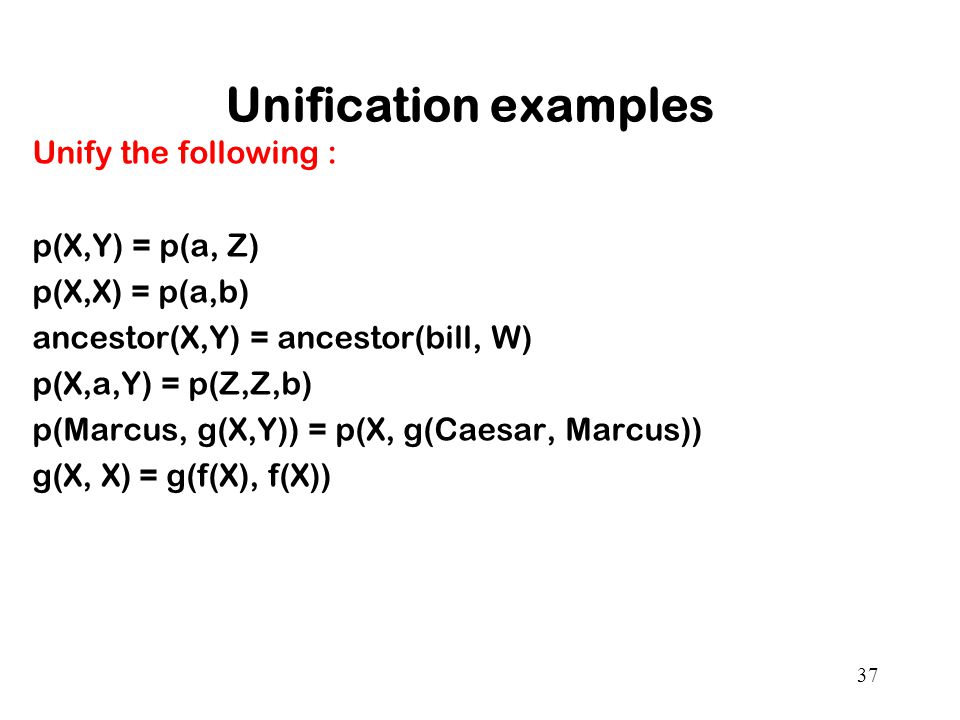
1 Logic Programming 2 A Little Bit Of Prolog Objects And Relations Between Objects Facts And Rules Upper Case Are Variables Parent Pam Bob Parent Tom Bob Ppt Download

2

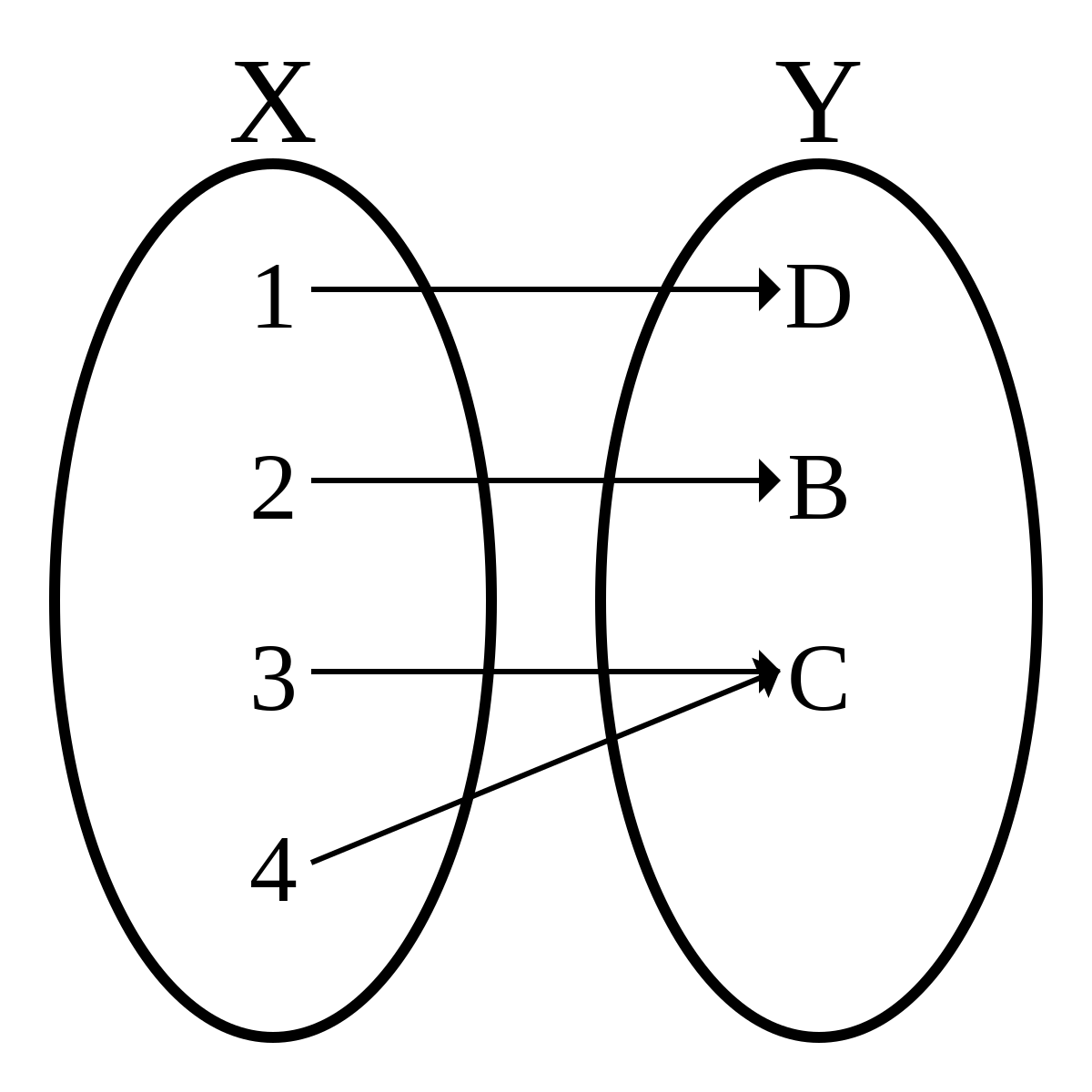
Surjective Function Wikipedia

Ejercicios Propuestos Normal

Tema 2

Problemas Resueltos 1oct Max

2

Groupoids An Overview Sciencedirect Topics

2

Bonner Zoologische Beitrage Zoologisches Herausgeber Und Forschungsinstitut Museo Koenig De Bonn La Biologia Zoologia La Especiacion De Los Microtus Savii Grupo 279 Lt Im N U El

Boc Cantabria Es

Useful Resources Bizagi Glossary

Nek Istanbul Edu Tr 4444

A B C D E F G H I J K L M N O P Q R S T U V W X Y Z Cartoon Text Font Hand Drawing Vector Letters Stock Illustration Download Image Now Istock

People Mpi Inf Mpg De

Bayesian Networks Ii What Bayesian Networks Are Good

Frontiers Effect Of Low Input Organic And Conventional Farming Systems On Maize Rhizosphere In Two Portuguese Open Pollinated Varieties Opv Pigarro Improved Landrace And Sinpre A Composite Cross Population Microbiology

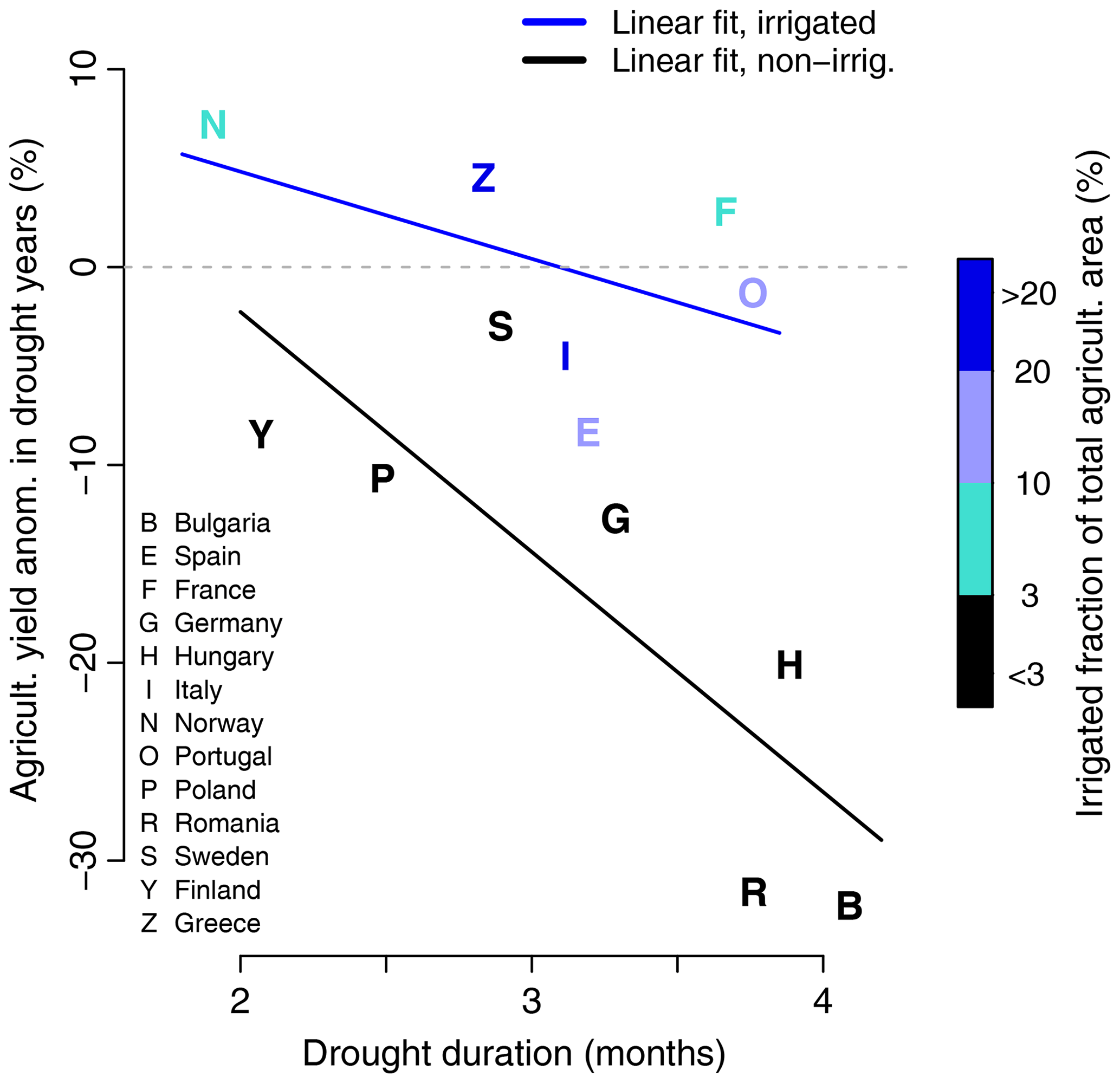
Bg Large Scale Biospheric Drought Response Intensifies Linearly With Drought Duration In Arid Regions

Sacitametam Com

Pdf Compactness Of Commutators Of Bilinear Maximal Calder O N Zygmund Singular Integral Operators

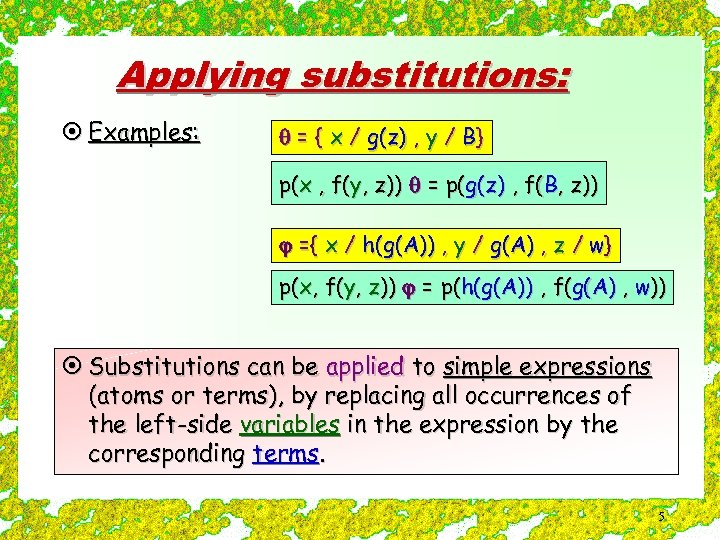
Ar For Horn Clause Logic Introducing Unification

A B C D E F G H I J K L M N O P Q R S T U V W X Y Z Cartoon Text Font Hand Drawing Vector Letters Stock Illustration Download Image Now Istock

The Crystal Structure Of K2ptcl4 And K2pdcl4 With Estimates Of The Factors Affecting Accuracy Mais 1972 Acta Crystallographica Section B Wiley Online Library

Shopping Special For Initial Bracelets R Also In U H Z C N Q Y P M T E A D B G S I J V K F L

A B C D E F G H I J K L M N O P Q R S T U V W X Y Z English Alphabet Capital Letters Pattern Of Gold Letters Vector Stock Il Stock Vector Illustration Of Text Letter

Solved 1 Write A Word Description Of Each Set A L B C Chegg Com

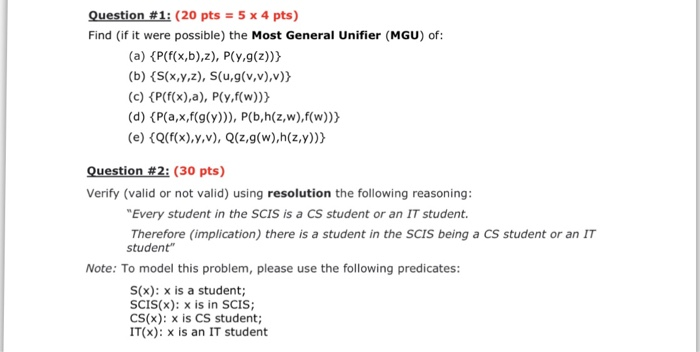
Solved Question 1 Pts 5x 4 Pts Find If It Were Chegg Com

Digital Csic Es

General Resolution Method In Fol Ppt Download

New Savings On Initial Bracelets M Also In D Z H K S Y B G A F X O P I W J C Q L N U R

1er Parcial Funciones

12 28 E Girls Live Dvd Blu Ray Disc We Girls Live 17 E G Evolution X L O I I T B V T N U O C T C G T F U C T E Girls Avexmobile

Spencerian Daily Practice Conclusion Z F G Y J B Handwriting

2

Solved Questions For Complex Analysis I Final Exam Math 5423 Docsity

2

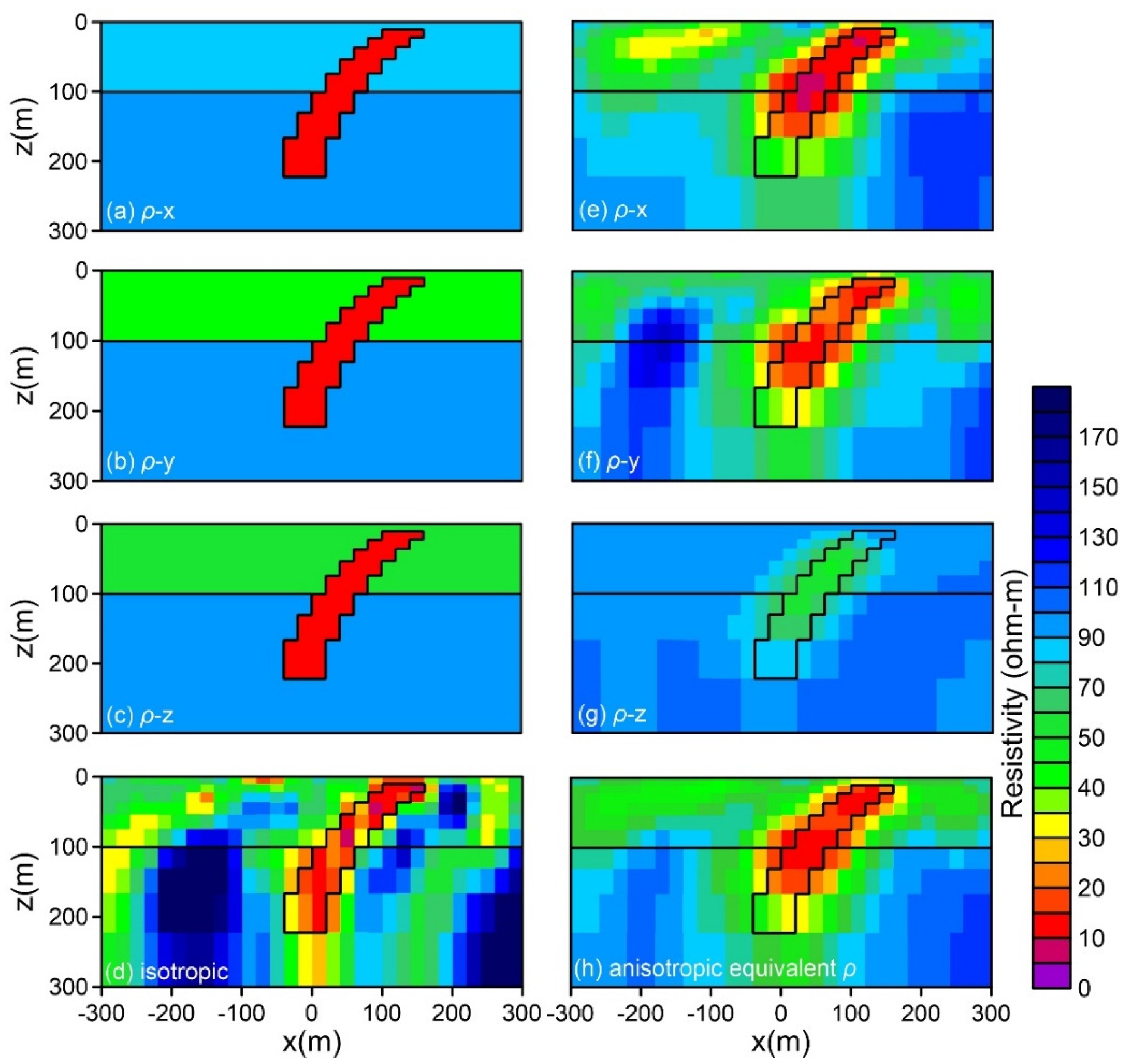
Minerals Free Full Text Three Dimensional Anisotropic Inversions For Time Domain Airborne Electromagnetic Data Html

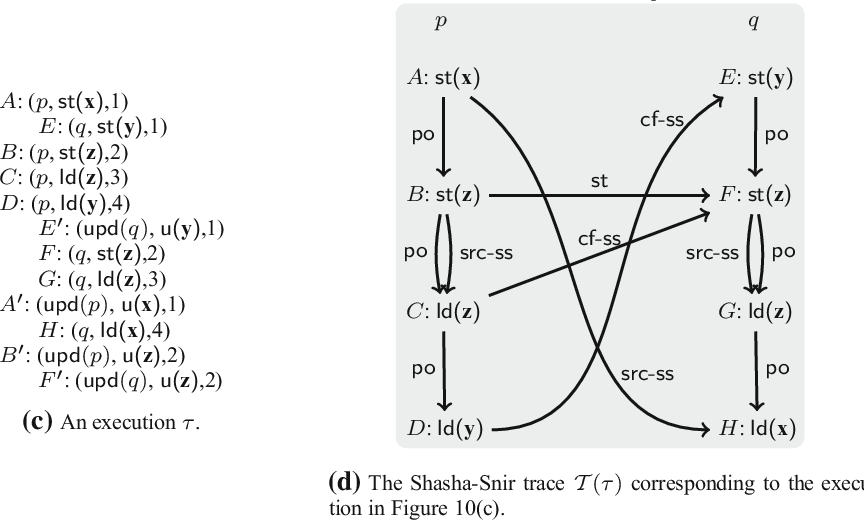
Pdf Stateless Model Checking For Tso And Pso Semantic Scholar

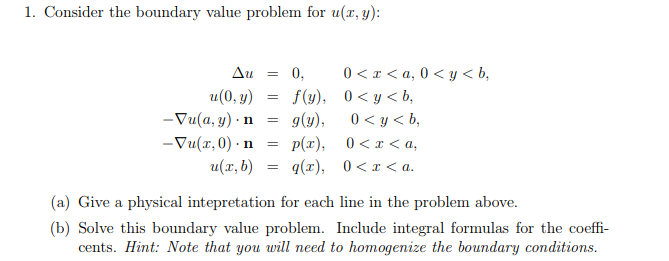
1 Consider The Boundary Value Problem For U R Y Chegg Com

Ocs Ciemat Es

Latest Singapore Airlines Krisflyer Accrual Roaming Alpaca

Shop Initial Bracelets T Also In E N F O L V Q S M R K U P C A X B G Z Y H I W D

Ejercicios Sobre Distribucion Normal Superprof

33 R P M Fotos E Imagenes De Stock Pagina 9 Alamy

2

2

Latin Letters A P Z B O E S M X D G U V W N H T R Q K F Y List Of Latin Letters Latin Letters Used In

2

The Predicted Genes In The Metagenome Were Matched To The Functional Download Scientific Diagram

Conjunto Ejercicios Y Teoria

Solved Find If It Were Possible The Most General Unifier Chegg Com

Q2 Unify If Possible The Following Pairs Of Chegg Com

Unstats Un Org

Departamento De Cs E Ingenieria De La Computacion Universidad Nacional Del Sur Pdf Free Download

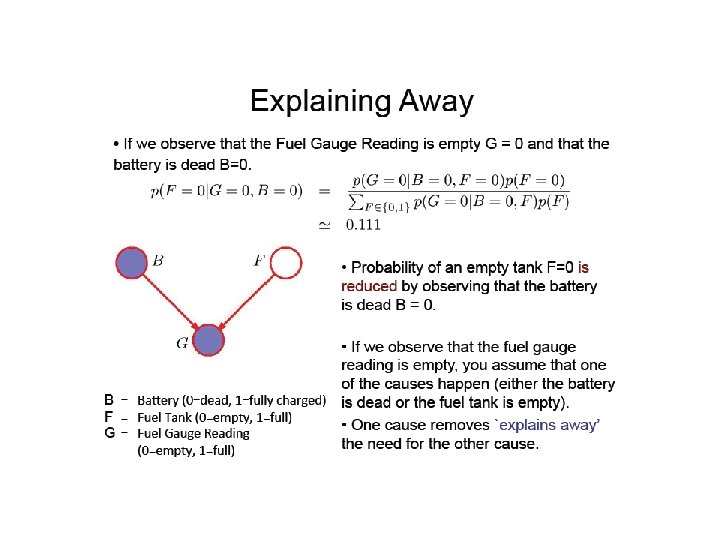
Bayesian Networks Ii What Bayesian Networks Are Good

Pr2 Plots A B C D E Laverania Plasmodia F G H I L M N Download Scientific Diagram

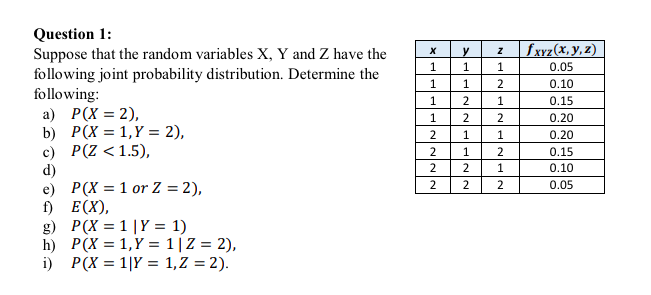
Answered Question 1 Suppose That The Random Bartleby

Ejercicios Sobre Distribucion Normal Superprof

Font Stylization Of The Letters Y And A B C D E F G H J K L M N O P Q R T V W S X Y Z Font

Futhark Runic Alphabet Runes Norse Runes

Guia Arboles Redes 17 Matematica Discreta Unsj Studocu

Ugr Es

A B C D E F G H I J K L M N O P Q R S T U V W X Y Z English Alphabet Capital Letters Pattern Of Letters Vector Stock Illustra Stock Vector Illustration Of Background Pattern